Speaker
Description
Physical and mathematical modeling is widely used to simulate cryoapplication processes. Mathematical modeling of this process [1,2] allows us to predict the temperature field of the frozen region. This makes it possible to determine the cryo-application time sufficient to destroy target cells and minimize damage to healthy cells under various experimental conditions. Moreover, simulation also predicts the depth of the cryoapplication impact, which can be difficult to measure in some situations in the living tissues. Then, we use temperature-dependent thermodynamical parameters of biological tissues to compare simulation with thermal imaging.
The problem with moving phase boundary is known as the Stefan problem. There are several ways to numerically solve that problem. One of them is gradually changing thermodynamic parameters close to the phase change boundary. This approach is effectively describing the freezing dynamics of a biological tissues. The appearance of solutes in water leads to change the freezing temperature in a range of temperatures around (-10C..-0.1C) due to change of solute concentration. By combining the usual thermal capacity with latent heat, we can define an effective thermal capacity. That allows us to solve the heat equation in 2D cylindrical geometry, see Fig. 1.
Generally thermodynamic properties of the biological tissues are highly dependent on temperature, so we have a non-uniform heat equation $\frac{\partial T}{\partial t}=\frac{1}{\rho C_\text{p}}\nabla k\nabla T$ . To solve this equation numerically, we use the finite differences method on a rectangular mesh to calculate the thermal balance of each node. Then we compare our results with thermal imaging of cryoapplication impact on rat skin [3].
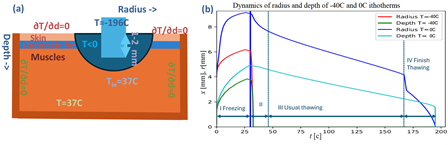
Fig. 1. (a) Principal scheme of the cryo-application problem for 2D cylindrical geometry with radial symmetry, where there is the cryo-applicator with a temperature of liquid Nitrogen pressed 1-2mm inside the soft tissues. (b) Dynamics of the maximum radius and depth of the ice spot for several isotherms. Typically consists of 4 phases, I-freezing, II-thawing thin layer of ice around the main ice spot, III-usual thawing, IV-finish thawing.
Acknowledgments: This research is sponsored by the National Research Foundation of Ukraine (Grant No. 2022.01/0094).
[1] M. Rossi and Y. Rabin, in: Proc. Int. Conf. "Modeling, Simulation & Visualization Methods" (MSV 2007), CSREA Press, 187-193.
[2] Y. Rabin and A. Shitzer, J. Biomech. Eng., 1997, 119, 146-152.
[3] G. Kovalev et al., Problems of Cryobiology and Cryomedicine, 2020 30, 359-368
