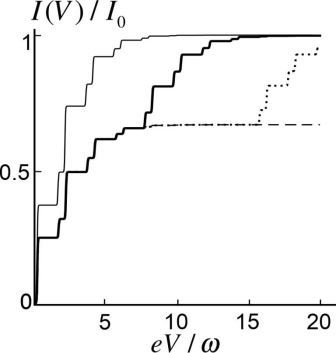
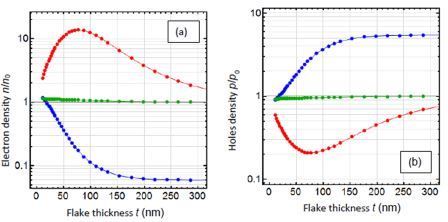
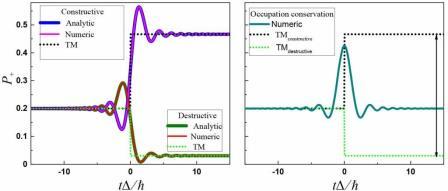
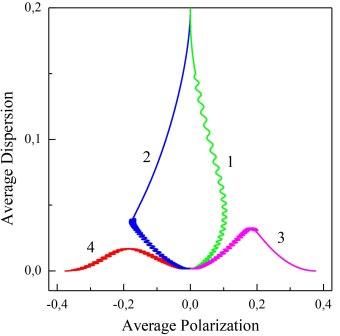
Ferroelectrics are among the most interesting objects for fundamental and applied studies of spontaneous polarization dynamics. Special efforts are intended to answer the question on how complex topological states such as flux-closure domains, polarization vortices, or skyrmions, which sometimes exist in nanosized ferroelectrics, can be controlled by elastic forces and/or electric fields.
Several authors [1-6] have studied numerically the electrocaloric effect (ECE) in ferroelectric nanoparticles using a phase field method combined with the Landau-Ginzburg-Devonshire (LGD) approach. These studies demonstrate the possibility to reveal a giant ECE in various ferroelectric nanoparticles, where the conditions for observing the effect are almost always determined in an empirical way, except for the case of single-domain nanoparticles [7]. Within the framework of the approach we explored the impact of the mismatch strain on ECE of core-shell ferroelectric nanoparticles with complex domain structure. We performed calculations for a multiaxial ferroelectric core covered with a paraelectric shell (see Fig. 1a), with or without mismatch strains. The latter are induced by the difference of the core and shell lattice constants. We studied the influence of the core radius on the electrocaloric cooling temperature and coercive field (see Fig. 1b). We revealed the significant asymmetry of the ferroelectric properties (transition temperature, polarization magnitude, coercive field) and ECE with respect to the sign of the mismatch strain. This result is in a qualitative agreement with experimental results of Barnakov et al. [8], who studied the ferroelectric properties of BaTiO3 nanocubes coated with metal carboxylates in two forms – one was crystalline and provided a lattice mismatch, and the other was non-crystalline without mismatch conditions. The revealed polar effects differed by many orders of magnitude for these two coatings. The analytical results obtained in this study can be used for the optimization of core-shell ferroelectric nanoparticle sizes for advanced applications in nanoelectronics and nano-coolers. Specifically, our results allow us to select optimal parameters to reach “giant” negative values of an electrocaloric response from an ensemble of non-interacting core-shell nanoparticles. A giant ECE, such as cooling by 20 K, could be very promising for advanced applications of ferroelectric nanocomposites in energy convertors and cooling systems.
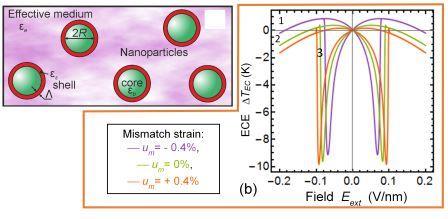
Figure 1. (a) Spherical ferroelectric NPs covered with a thin semiconducting shell and placed in an isotropic dielectric polymer (b) Dependence of the EC temperature change $\Delta T_EC$ on a quasi-static external electric field for nanoparticles with a $BaTiO_3$ core covered with a rigid shell $ε_s$=300. The curves are calculated for different values of mismatch strain between the core and shell $u_m$ = –0,4% (curve 1), 0 (curve 2), and +0.4% (curve 3). Shell thickness $\Lambda$ = 4 nm, and T = 293 K.
Acknowledgements. A.N.M. acknowledges EOARD project 9IOE063 and related STCU partner project P751. R.H. acknowledges funding from the French National Research Agency through contract ANR-18-CE92-0052 “TOPELEC”. V.Y.R. acknowledges the support of COST Action CA17139.
[1] X. Chen, and C. Fang. Study of electrocaloric effect in barium titanate nanoparticle with core–shell model. Physica B: Condensed Matter 415, 14 (2013).
[2] B. Li, J. B. Wang, X. L. Zhong, F. Wang, Y. K. Zeng, and Y. C. Zhou. Giant electrocaloric effects in ferroelectric nanostructures with vortex domain structures. RSC Advances 3, 7928 (2013).
[3] Y. K. Zeng, B. Li, J. B. Wang, X. L. Zhong, W. Wang, F. Wang, and Y. C. Zhou. Influence of vortex domain switching on the electrocaloric property of a ferroelectric nanoparticle. RSC Advances 4, 30211 (2014).
[4] Z. Y. Chen, Y. X. Su, Z. D. Zhou, L. S. Lei, and C. P. Yang. The influence of the electrical boundary condition on domain structures and electrocaloric effect of PbTiO3 nanostructures. AIP Advances 6, 055207 (2016).
[5] F. Wang, L. F. Liu, B. Li, Y. Ou, L. Tian, and W. Wang. Inhomogeneous electric-field–induced negative/positive electrocaloric effects in ferroelectric nanoparticles. EPL (Europhysics Letters) 117, 57002 (2017).
[6] C. Ye, J. B. Wang, B. Li, X. L. Zhong, Giant electrocaloric effect in a wide temperature range in PbTiO3 nanoparticle with double-vortex domain structure. Sci. Rep. 8, 293 (2018).
[7] A. N. Morozovska, E. A. Eliseev, M. D. Glinchuk, H. V. Shevliakova, G. S. Svechnikov, M. V. Silibin, A. V. Sysa, A. D. Yaremkevich, N. V. Morozovsky, and V. V. Shvartsman. Analytical description of the size effect on pyroelectric and electrocaloric properties of ferroelectric nanoparticles. Phys. Rev. Materials 3, 104414 (2019).
[8] Yu. A. Barnakov, I. U. Idehenre, S. A. Basun, T. A. Tyson, and D. R. Evans. Uncovering the Mystery of Ferroelectricity in Zero Dimensional Nanoparticles. Royal Society of Chemistry, Nanoscale Adv. 1, 664 (2019).
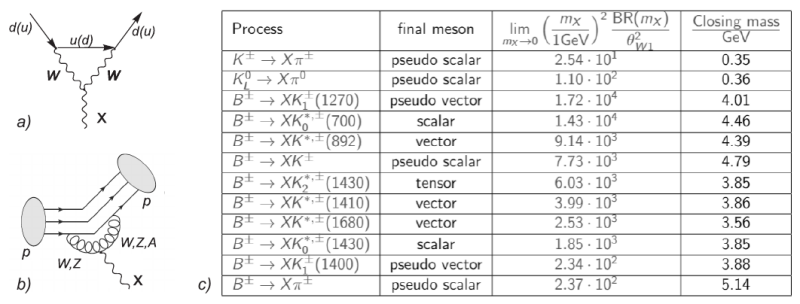 Figure 1. a) loop diagram of quarks interactions with CS particles; b) diagram of CS production in meson's decay c) decay of $B$- and $K$-mesons with CS production.
Figure 1. a) loop diagram of quarks interactions with CS particles; b) diagram of CS production in meson's decay c) decay of $B$- and $K$-mesons with CS production.